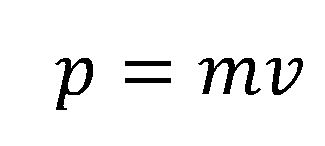Conservation of Momentum

If we consider Newton's 3rd law, "For every action there is an equal and opposite reaction", it can also be said that forces occur in pairs.  If we consider the 2 skaters, we can see that their momentum is zero, and when they are added together, it equals zero.

The skaters both push and so according to Newtons 3rd law, equal and opposite forces will apply

Now because they exert the force on each other for the came time we can say:

which can be rewritten as:

Rearranging we get:

Which in turn leads to the conclusion:

This makes perfect sense when you remember that momentum is a vector quantity.  Therefore if one object is moving in the opposite direction relative to the other, its value will be negative.

In the scenarios presented to you, it will often involve 2 cars colliding in one form or another.  There are a number of possibilities that may happen as a result of these collisions.

1. The case lock together (in this case we treat them as a single object with mass of the 2 cars combined)
2. The cars are stationary
3. The cars may bounce off each other and move in the opposite direction.

Momentum

Momentum can be described as "mass in motion". Therefore it is proportional to  both the mass of the object and its velocity.  Put simply:

Where p is momentum, m is mass and v is velocity.  Momentum is a vector quantity and has the units Newton seconds.  Momentum can also be defined with a slight alteration to Newton's 2nd Law.

Take:

The relationship between an object's mass m, its acceleration a, and the applied force F is F = ma

alter to:

The rate of change of momentum is directly proportional to the magnitude of the net force and the direction of the net force.

F = ma  can be rewritten as:

which can be rewritten as:

Impulse is known as change in momentum.  Impulse can also be written as below:

From this point, we will move onto conservation of momentum. 

Conservation of Energy

There are plenty of examples of the conservation of energy.  This post will simply look at the changes of gravitational potential energy and kinetic energy.

Within a closed system the amount of energy remains constant and energy is neither created nor destroyed. Energy can be converted from one form to another (potential energy can be converted to kinetic energy) but the total energy within the system remains fixed.

Let's use the example above.  The ball on a frictionless pendulum will swing forever.  However, the energy is converted repeatedly as the ball swings back and forth.  At the highest point of the swing, it stops moving for an instant. Kinetic energy is therefore zero, and because its at its highest point, the potential energy is greatest.  At the bottom of the swing when the pendulum can go no lower, the kinetic energy is greatest and the potential energy is at its lowest.  Therefore we can see that the energy has been converted.

An example that we studied in the lab was the falling object through the light gates at certain heights.  Some groups found that nearly all of the calculated gravitational potential energy had been converted to kinetic energy which was measured and calculated.

A great example of conversions of energy is a hydro electric dam.  Here we have potential energy being stored behind the wall of the dam, which is converted to kinetic energy when travelling down the penstock and the turbine and then electrical energy at the generator.  

Kinetic and Potential Energy

Kinetic energy refers to the amount of energy a moving object has.  The greater the mass and the greater the kinetic energy. 

The formula for kinetic energy is:

The units of energy are Joules (J).  It is a scalar, not a vector quantity.

This also means that the faster an object travels, the amount of energy will increase exponentially. This is one of the many reasons that we have low speed zones on certain areas such as school.

Similarly, the more massive an object is, the more kinetic energy it has if it is travelling at the same velocity as a smaller one. 



Another concept you need to be aware of is the idea of work.  Work is done when a force is applied to move an object a certain distance.  So for our friend below , he has to overcome friction to get the box to move.  He will also have to push it the entire distance it has to move.  The formula for work is:

Not only does work involve moving objects, but also with moving ones.  You should be aware that a force will cause an object to undergo acceleration.

As a result, the force applied over a certain distance will result in a change in kinetic energy.  Thus:

where u = the initial velocity and v = the final velocity.

Another way in which work can be done is from lifting an object off the ground.  The work done is turned into gravitational potential energy.  Recall that F=mg for falling objects and displacement can also mean height.  Therefore:

 The implication is that the gravitational potential energy will be converted to kinetic energy is a lifted object is allowed to fall.  This will be the subject of the next post.